(x-y)^3 expand formula 128271

The calculator allows you to expand and collapse an expression online , to achieve this, the calculator combines the functions collapse and expand For example it is possible to expand and reduce the expression following ( 3 x 1) ( 2 x 4), The calculator will returns the expression in two forms expanded and reduced expression 4 14 ⋅ xYou can put this solution on YOUR website!Want to solve the equation a 2(x)y′′ a 1(x)y′ a 0(x)y = f(x) (61) subject to boundary conditions We can write such an equation in operator form by defining the differential operator L = a 2(x) d2 dx2 a 1(x) d dx a 0(x) Then, Equation (61) takes the form Ly = f As we saw in the general boundary value problem (4) in Section 432,

What Is The Expansion Of A B C 3 Quora

(x-y)^3 expand formula

(x-y)^3 expand formula-All Steps Visible Step 1 Third term Step 1 Answer $$a_ {3} =\left (\frac {5!} {2!3!} \right)\left (a^ {3} \right)\left (\sqrt {2} \right)^ {2} $$ Step 2 Expand the coefficient Step 2 Answer $$a_ {3} =\left (\frac {4\times 5\times 3!} {2\times 3!} \right)\left (a^ {3} \right)\left (\sqrt {2} \right)^ {2} $$Stack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange

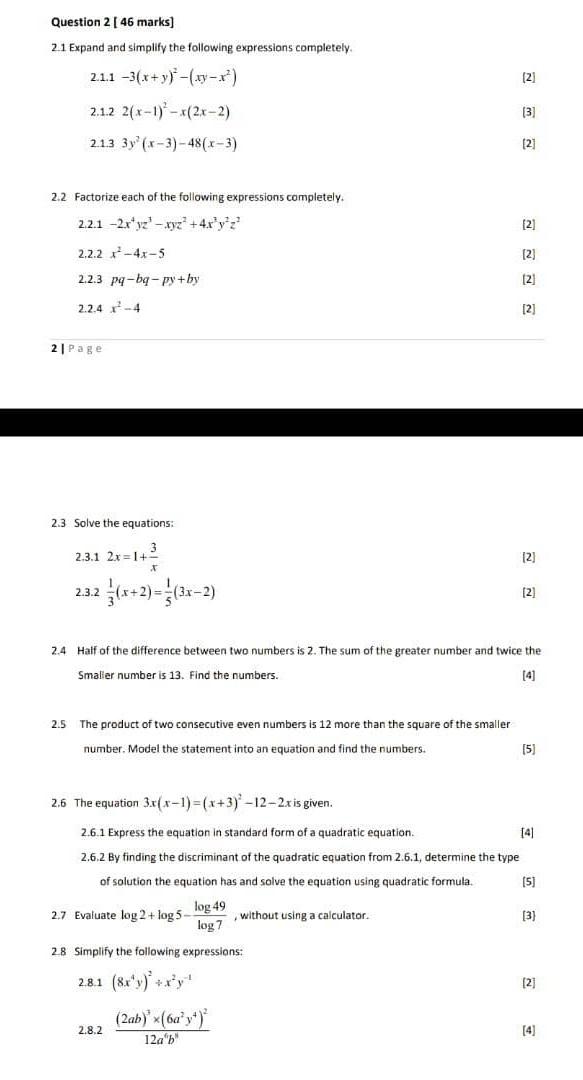
Solved Question 2 46 Marks 2 1 Expand And Simplify The Chegg Com

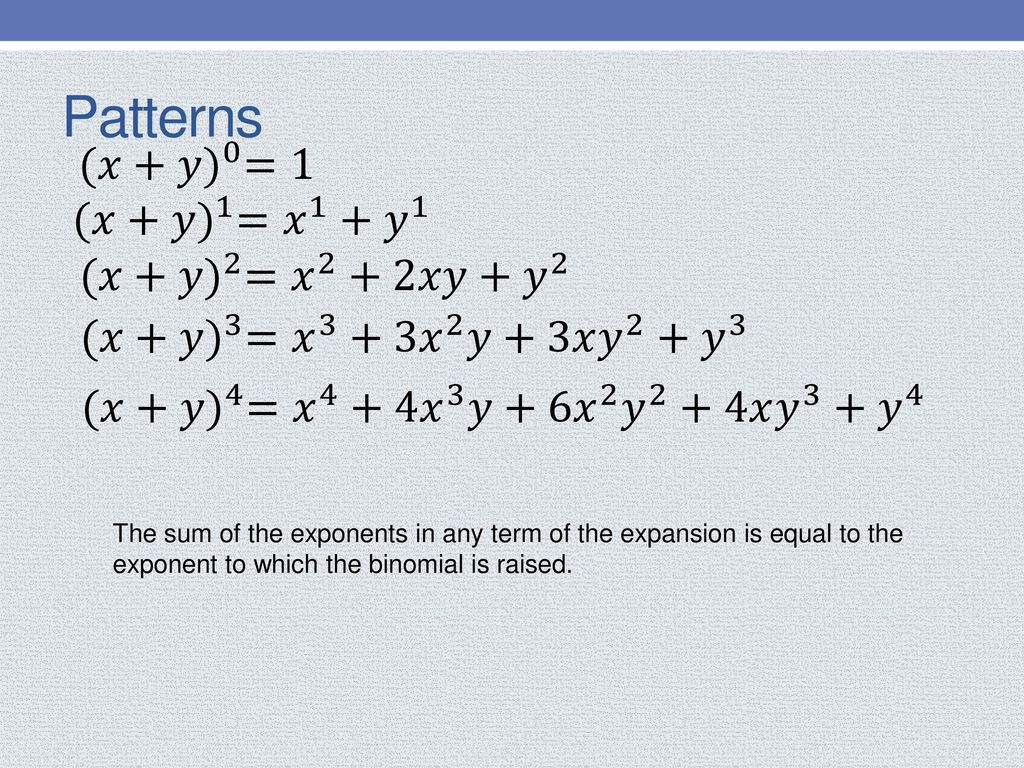
 We know that (xy) 3 can be written as (xy)(xy)(xy) We know that (xy)(xy) can be multiplied and written as x 2xy yx y 2 (xy) = x 22xy y 2 (xy) = x 32x 2 y xy 2yx 2 2xy 2y 3 = x 33x 2 y 3xy 2y 3 Answer (xy) 3 =x 33x 2 y 3xy 2y 3= 2 ⋅ 1 = 2, 1!Key Takeaways Key Points According to the theorem, it is possible to expand the power latex(x y)^n/latex into a sum involving terms of the form latexax^by^c/latex, where the exponents latexb/latex and latexc/latex are nonnegative integers with latexbc=n/latex, and the coefficient latexa/latex of each term is a specific positive integer depending on latexn/latex

243x 5 810x 4 y 1080x 3 y 2 7x 2 y 3 240xy 4 32y 5 Finding the k th term Find the 9th term in the expansion of (x2y) 13 Since we start counting with 0, the 9th term is actually going to be when k=8 That is, the power on the x will 138=5 and the power on the 2y will be 8 The binomial expansion formula is given by This is also known as the binomial theorem formula A simple binomial formula is obtained by substituting 1 for y so that it involves a single variable In this form, the formula becomes An example to prove the binomial formula (xy) 3 = (xy) (xy) (xy) =xxxxyxxxyxyyyxxyxyyyxyyyThe first factor of the product is a sum containing 2 terms The first term of the sum is equal to X The second term of the sum is equal to Y The second factor of the product is equal to a sum consisting of 2 terms The first term of the sum is equal to X The second term of the sum is equal to negative Y open bracket X plus Y close bracket multiplied by open parenthesis X plus negative Y

If a binomial expression (x y) n is to be expanded, a binomial expansion formula can be used to express this in terms of the simpler expressions of the form ax by c in which 'b' and 'c' are non negative integers The value of 'a' completely depends on the value of 'n' and 'b'Answer (1 of 16) Mentally examine the expansion of (xyz)^3 and realize that each term of the expansion must be of degree three and that because xyz is cyclic all possible such terms must appear Those types of terms can be represented by x^3, x^2y and xyz If x^3 appears, so must y^3 and z^3It is read as x plus y whole cube It is mainly used in mathematics as a formula for expanding cube of sum of any two terms in their terms ( x y) 3 = x 3 y 3 3 x 2 y 3 x y 2

How Can We Expand X Y 1 2 Youtube

Binomial Expansion Combinatorics Video Khan Academy

= x 3 y 3 z 3 3 (x y) (y z) (z x) 0 ⇒ (x y z) 3 = x 3 y 3 z 3 3 (x y) (y z) (z x), in the form involving factors Remark The above result can be simplified (more precisely, expanded) further, as under We therefore consider the following expression, 3 (x y) (y z) (z x) = 3 (x y) y (z x) z (z x) = 3 (x y) (y z y x z 2 z x), after multiplication = 3 x (y( n − k)!Harnett, ch 3) A The expected value of a random variable is the arithmetic mean of that variable,

A B N And A B N Formula Expander

What Is The Expansion Of A B C 3 Quora

= 1 and 0!For jyj < 1 Formula (1) leads toSo, let's try to derive the identity x 3 y 3 using the identity for (x y) 3 Rearranging the terms in the expansion, we will get our identity for x 3 y 3 Substituting the values of x and y in the formula, Thus, we have factorized a given polynomial using our algebraic identity

Exponent Rules 7 Key Strategies To Solve Tough Equations Prodigy Education

ベスト X5 Y5 Expand シモネタ

The calculator can also make logarithmic expansions of formula of the form ln ( a b) by giving the results in exact form thus to expand ln ( x 3), enter expand_log ( ln ( x 3)) , after calculation, the result is returned The calculator makes it possible to obtain the logarithmic expansionThis calculator can be used to expand and simplify any polynomial expressionSolution The expansion is given by the following formula ( a b) n = ∑ k = 0 n ( n k) a n − k b k, where ( n k) = n!

Expand 1 X Y 3 Whole Cube Studyrankersonline

General Expanded Form Of X Y Z K Mathematics Stack Exchange

An outline of Isaac Newton's original discovery of the generalized binomial theorem Many thanks to Rob Thomasson, Skip Franklin, and Jay Gittings for their👉 Learn all about sequences In this playlist, we will explore how to write the rule for a sequence, determine the nth term, determine the first 5 terms orLearn about expand using our free math solver with stepbystep solutions

Tutorial 54 The Binomial Theorem

Learn Algebraic Identity Of X Y And X Y In 3 Minutes

 For example, if we want to confirm that x=6 is a solution to the equation x 3 − 3 x 2 − 16 x = 12 the following Mathematica command accomplishes this Simplify x^3 3 x^2 16 x == 12, x == 6 Out 15= True The Expand command expands a function or an equation Note this is when in the above expansion formula a=x^2 and the x is really y^2 so we do (x^2y^2) in that order Last edited One test is worth a thousand expert opinions, but one expert specification is worth a thousand tests Mathematics is the shortcut to understanding natureExpandcalculator en Related Symbolab blog posts Middle School Math Solutions – Equation Calculator

Section 8 5 The Binomial Theorem Ppt Download

What Is The Answer Of X Y Quora

 #(xy)^3=(xy)(xy)(xy)# Expand the first two brackets #(xy)(xy)=x^2xyxyy^2# #rArr x^2y^22xy# Multiply the result by the last two brackets #(x^2y^22xy)(xy)=x^3x^2yxy^2y^32x^2y2xy^2# #rArr x^3y^33x^2y3xy^2#The properties of logarithms formulas that are applied for logarithmic calculations are given below 1 x m = a ⇒ log x a = m 2 log a 1 = 0 3 log a a = 1 4 log a ( x y) = log a x log a y 5 log a x y = log a x – log a y 6 log a ( x m) = m log a x 7 log a x = log c x log c a 8 a log3 4 5 6 7 8 9 0, < > ≤ ≥ ^ √ ⬅ F _ ÷ (* / ⌫ A ↻ x y = G

ベスト X5 Y5 Expand シモネタ

Learn Algebraic Identity Of X Y And X Y In 3 Minutes

75 Matrices and Matrix Operations;(3 − 0)!(0!) = 3!73 Systems of Nonlinear Equations and Inequalities Two Variables;

Binomial Theorem Protonstalk

Make X The Subject Gcse Maths Steps Examples Worksheet

Firstly observe the pattern of the numbers whether the numbers have whole ^3 as power or not and in the form of (x1)^3 Write down the formula of (x 1) 3 (x 1) 3 = x 3 3x 2 x 1 Substitute the values in the (x 1) 3 formula and simplify Make your child naturally math mindedIn elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomialAccording to the theorem, it is possible to expand the polynomial (x y) n into a sum involving terms of the form ax b y c, where the exponents b and c are nonnegative integers with b c = n, and the coefficient a of each term is a specific positiveFactor x^3y^3 x3 − y3 x 3 y 3 Since both terms are perfect cubes, factor using the difference of cubes formula, a3 −b3 = (a−b)(a2 abb2) a 3 b 3 = ( a b) ( a 2 a b b 2) where a = x a = x and b = y b = y (x−y)(x2 xyy2) ( x y) ( x 2 x y y 2)

1

3 Expand With The Help Of Formula X Y 3 2

(x y) 5 (x – y) 5 = 25C 0 x 5 5C 2 x 3 y 2 5C 4 xy 4 = 2(x 5 10 x 3 y 2 5xy 4) Now (√2 1) 5 (√2 − 1) 5 = 2(√2) 5 10(√2) 3 (1) 2 5(√2)(1) 4 =58√2 Binomial Expansion Important points to remember The total number of terms in the expansion of (xy) n are (n1) The sum of exponents of x and y is always nGet stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!(c) h(x)= 1 2x3;

The Binomial Theorem Binomial Expansions Using Pascal S Triangle Subsets

Expand And Simplify Binomial Squares 2x 3y 2 Youtube

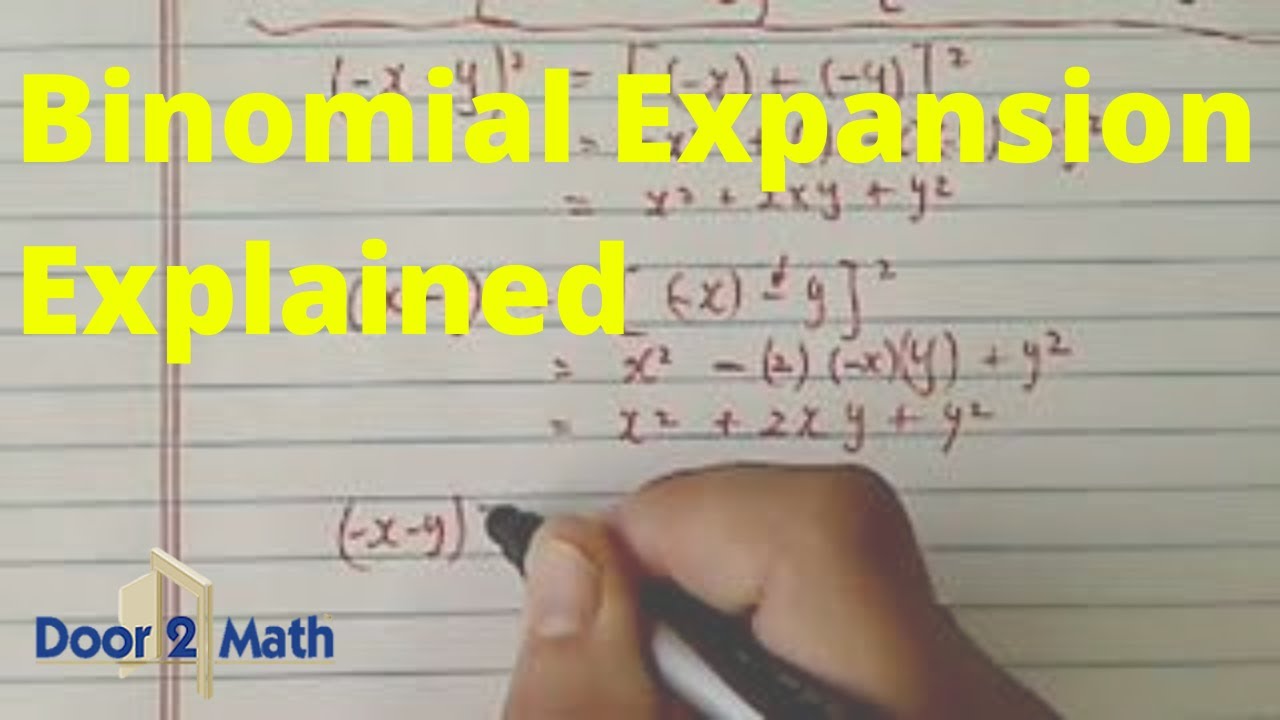
Binomial Theorem Examples Example 1 Expand the given equation (x/2 3/y)4 Sol Using the equation, ( x y) n = ∑ i = 0 n n C r x n − r y r , we get x 2 3 y) 4 = 4 C 0 ( x 2) 4 4 C 1 ( x 2) 3 ( 3 y) 4 C 2 ( x 2) 2 ( 3 y) 2 4 C 3 ( x 2) ( 3 y) 3 4 C 4 ( 3 y) 4Trigonometry Expand (xy)^3 (x y)3 ( x y) 3 Use the Binomial Theorem x3 3x2y3xy2 y3 x 3 3 x 2 y 3 x y 2 y 3(b) g(x)= x x2;

What Is The Taylor Series Expansion Of Math E X Math About Zero Quora

Ml Aggarwal Solutions For Class 9 Chapter 3 Expansions Download Free Pdf

How do you expand an equation into general form such as y=2 (x3)^28 y=2 (x3)^28 (1) (y8) = 2 (x3)^2 Squaring both the sides (y8)^2 = 2 (x3)^2^2 y^216y64 = 2^2 (x3)^2^2 (using (ab)^2 = a^2 2abb^2 formula on the LHS where a = y and b = 8)Identify binomial coefficients given the formula for a combination Expand a binomial using the binomial theorem Use the binomial to find a single term in a binomial Previously, we studied combinations In the shortcut to finding latex{\left(xy\right)}^{n}/latex, we will need to use combinations to find the coefficients that will appear= 1 _ 3C0 = 3!

A B Formula Identity

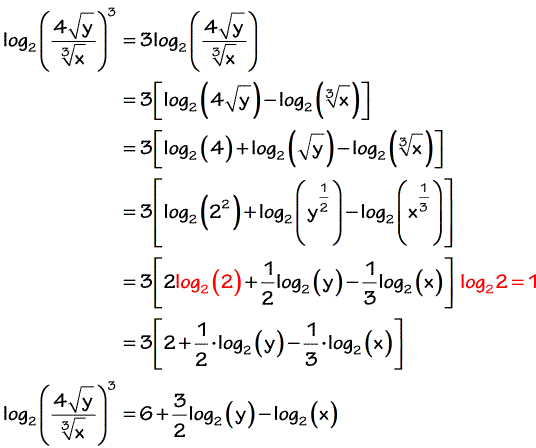
Expanding Logarithms Chilimath

Expectations Expectations (See also Hays, Appendix B;= 3 ⋅ 2 ⋅ 1 = 6, 2!A =1 Solution (a) We shall use (1) by &rst rewriting the function as follows 1 1x2 1 1¡(¡x2) y==¡x2 1 1¡y = X1 n=0 yn;

Using The Binomial Theorem College Algebra

Solved 2 Use The Rules Of Logs To Expand Each Of The Chegg Com

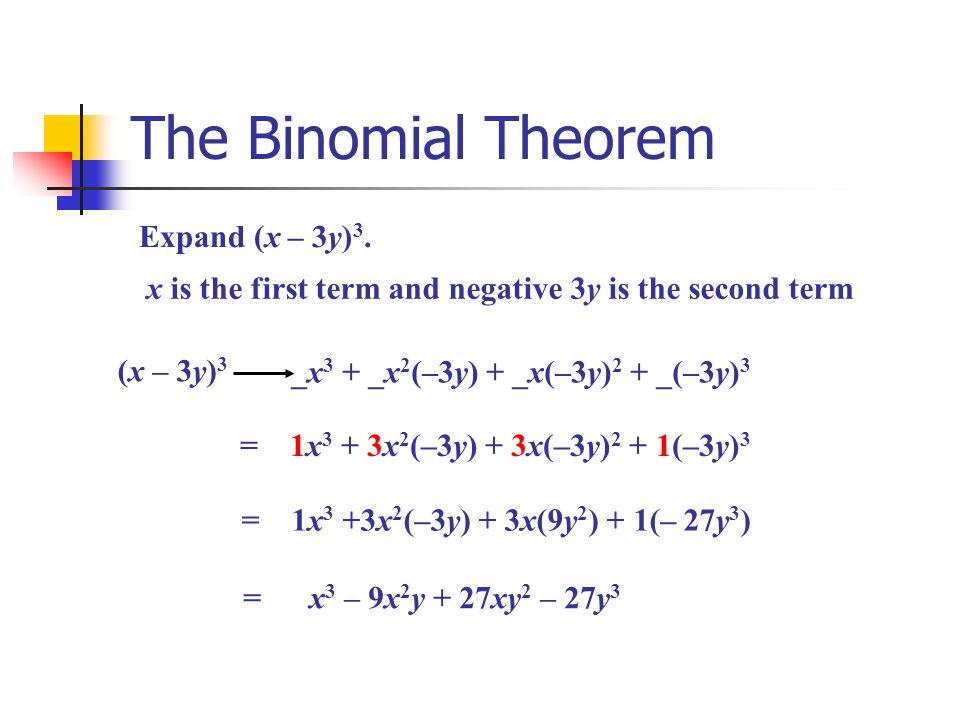
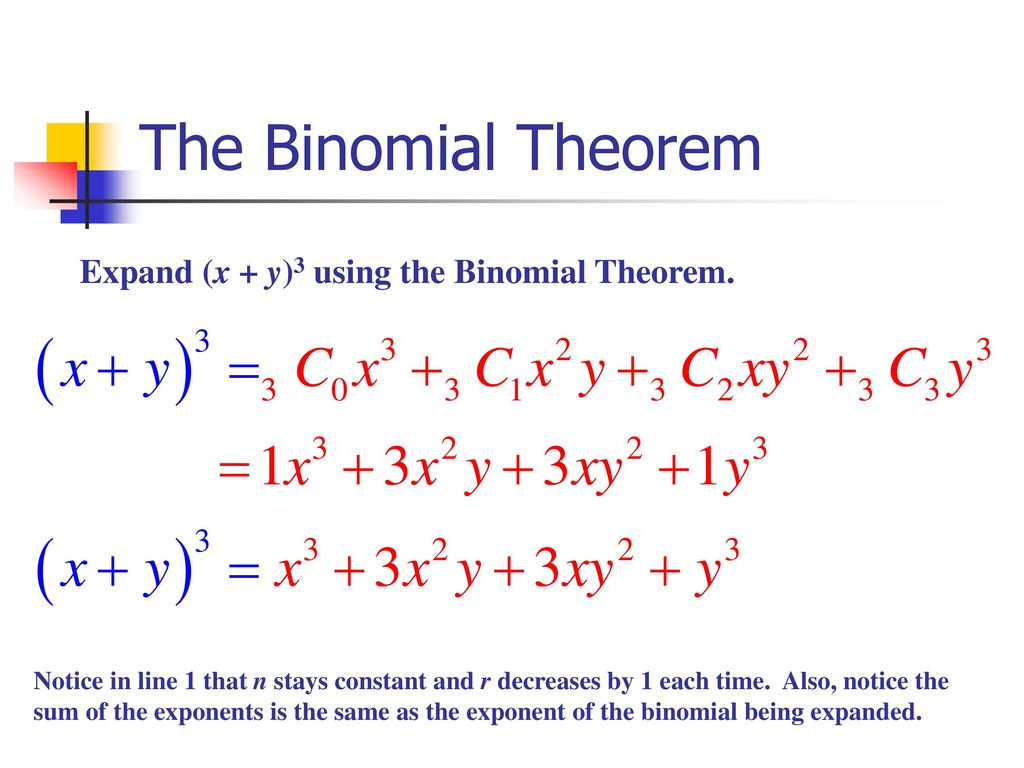
In the expansion of (5 x 3 y) n, (5 x 3 y(x y) 3 = x 3 3x 2 y 3xy 2 y 3 In general the expansion of the binomial ( x y ) n is given by the Binomial Theorem Theorem 671 The Binomial Theorem top Explanation Use the Binomial expansion (note the exponents sum to the power in each term) (x y)3 =3 C0x3y0 3 C1x2y1 3 C2x1y2 3 C3x0y3 Remember 3!

Expanding Polynomial Factors Maths First Institute Of Fundamental Sciences Massey University

4 The Binomial Theorem

Algebra Calculator is a calculator that gives stepbystep help on algebra problems See More Examples » x3=5 1/3 1/4 y=x^21 Disclaimer This calculator is not perfect Please use at your own risk, and please alert us if something isn't working Thank you= 1 ⋅ 2 ⋅ ⋅ n We have that a = 2 x, b = 5, and n = 3 Therefore, ( 2 x 5) 3 = ∑ k = 0 3 ( 3 k) ( 2 x) 3 − k 5 k Now, calculate the product for every value of k from 0 to 3 Thus, ( 2Free expand & simplify calculator Expand and simplify equations stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy Learn more expand\(x^23y)^3;

Binomial Coefficients And The Binomial Theorem

How To Expand Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube

Cube of difference (x y) 3 = x 3 3x 2 y 3xy 2 y 3So to find the expansion of (x − y) 3, we can replace y with (− y) in (x y) 3 = x 2 3 x 2 y 3 x y 2 y 3 This is the required expansion for ( x − y ) 3 Let's now use these identities toExamples on (a b)^3 Formula Example 1 Solve the following expression using (a b) 3 formula (2x 3y) 3 Solution To find (2x 3y) 3 Using (a b) 3 Formula, (a b) 3 = a 3 3a 2 b 3ab 2 b 3 = (2x) 3 3 × (2x) 2 × 3y 3 × (2x) × (3y) 2 (3y) 3 = 8x 3 36x 2 y 54xy 2 27y 3 Answer (2x 3y) 3 = 8x 3 36x 2 y 54xy 2 27y 3 Example 2 Find the value of x 3 y 3 if

Factorials To Binomial Theorem

Expanding Three Terms

76 Solving Systems with Gaussian Elimination;3) Solve the equation x 2 25 = 0 Solution x 2 25 = (x 5)(x 5) => we have to solve the following 2 equations x 5 = 0 or x 5 = 0 so the equation have two decisions x = 5 and x = 5 Related Resources Polynomial identities quiz Simplifying polynomial expressions problems with solutions Factoring polynomials problems withExpand Master and Build Polynomial Equations Calculator Expand Expresions Write Equation with Roots < Enter Expression < Enter Roots Expand (2x 5) 3 Since (2x 5) 3 is a binomial expansion, we can use the binomial theorem to expand this expression The formula for this is a (x y) n = aΣ k = 0 to n C (n,k) x nk y k where C (n

How To Expand The Binomial X Y 10 Quora

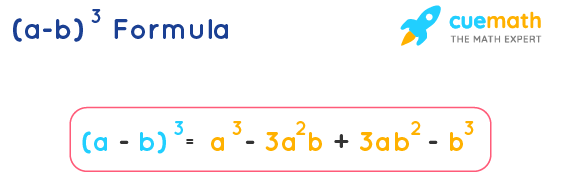
A Minus B Whole Cube Formula Examples A B 3 Formula

The primary example of the binomial theorem is the formula for the square of xy The coefficients 1, 2, 1 that appear in this expansion are parallel to the 2nd row of Pascal's triangle (Because the top "1" of the triangle is row 0) The coefficients of higher powers of x y on the other hand correspond to the triangle's lower rowsExpand (S) multiplies all parentheses in S, and simplifies inputs to functions such as cos (x y) by applying standard identities expand (S,Name,Value) uses additional options specified by one or more namevalue pair arguments For example, specifying 'IgnoreAnalyticConstraints' as true uses convenient identities to simplify the inputCircle on a Graph Let us put a circle of radius 5 on a graph Now let's work out exactly where all the points are We make a rightangled triangle And then use Pythagoras x 2 y 2 = 5 2 There are an infinite number of those points, here are some examples

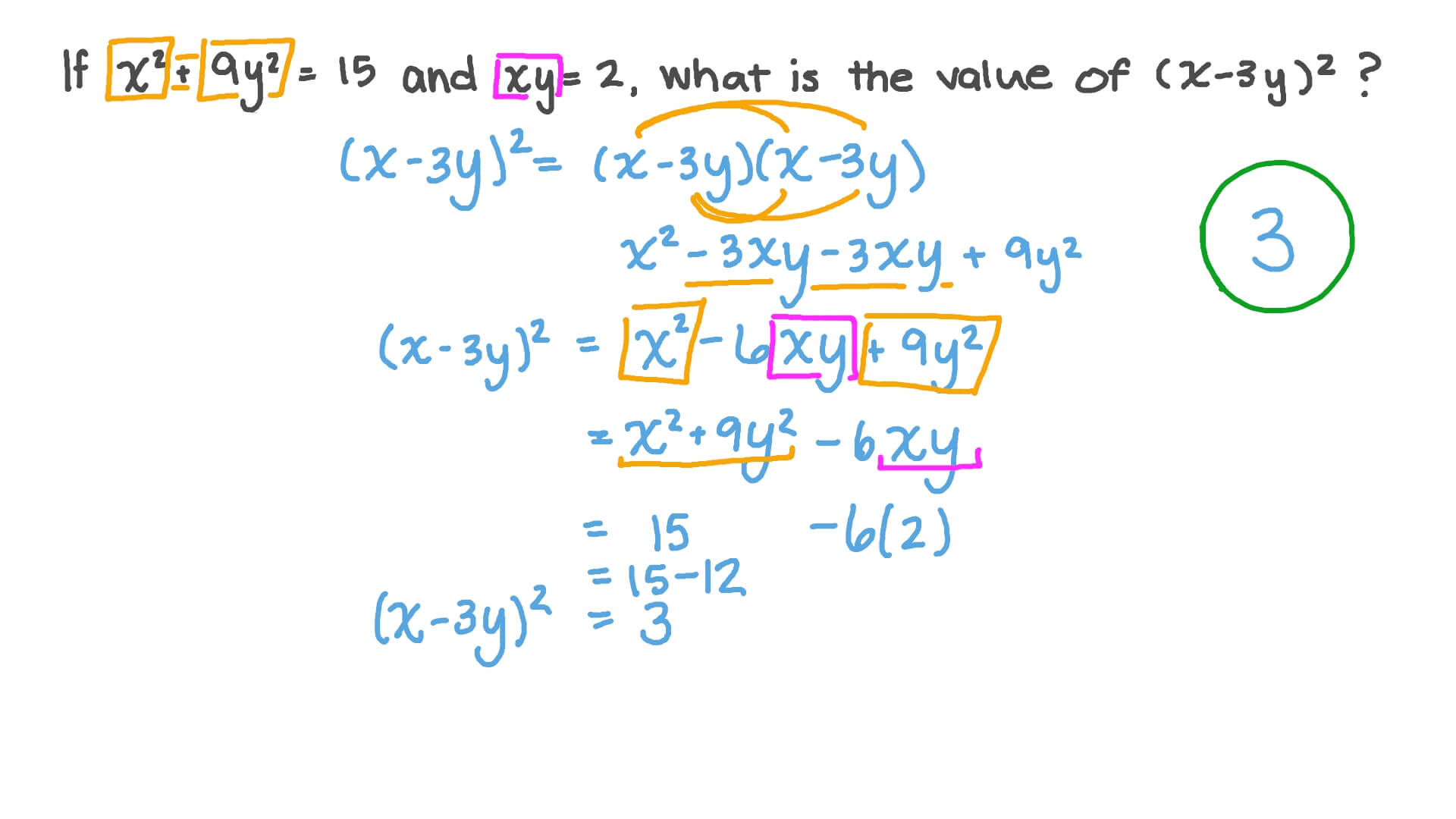
Question Video Evaluating Algebraic Expressions Using Algebraic Identities Nagwa

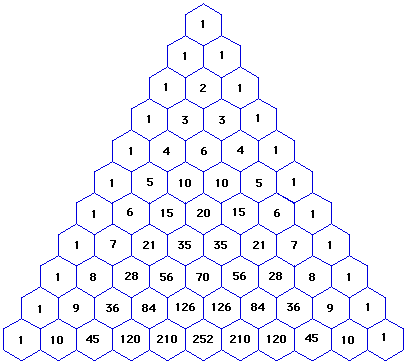
How Do You Expand X Y 10 Socratic

72 Systems of Linear Equations Three Variables;

Baker Campbell Hausdorff Formula Wikipedia

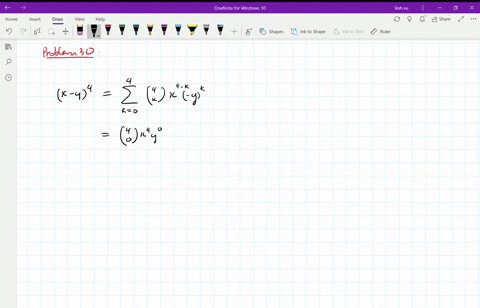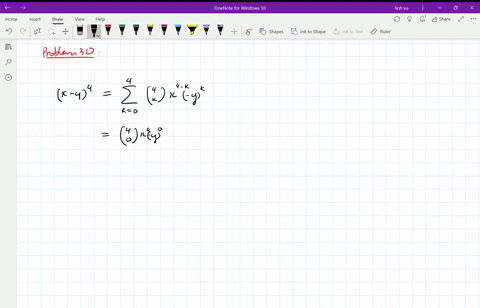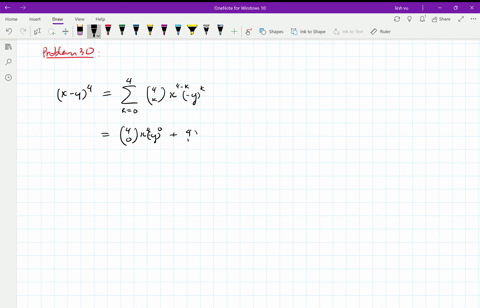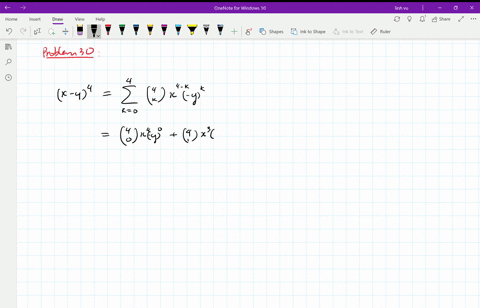
Solved Expand The Binomial Using The Binomial Formula X 2 4

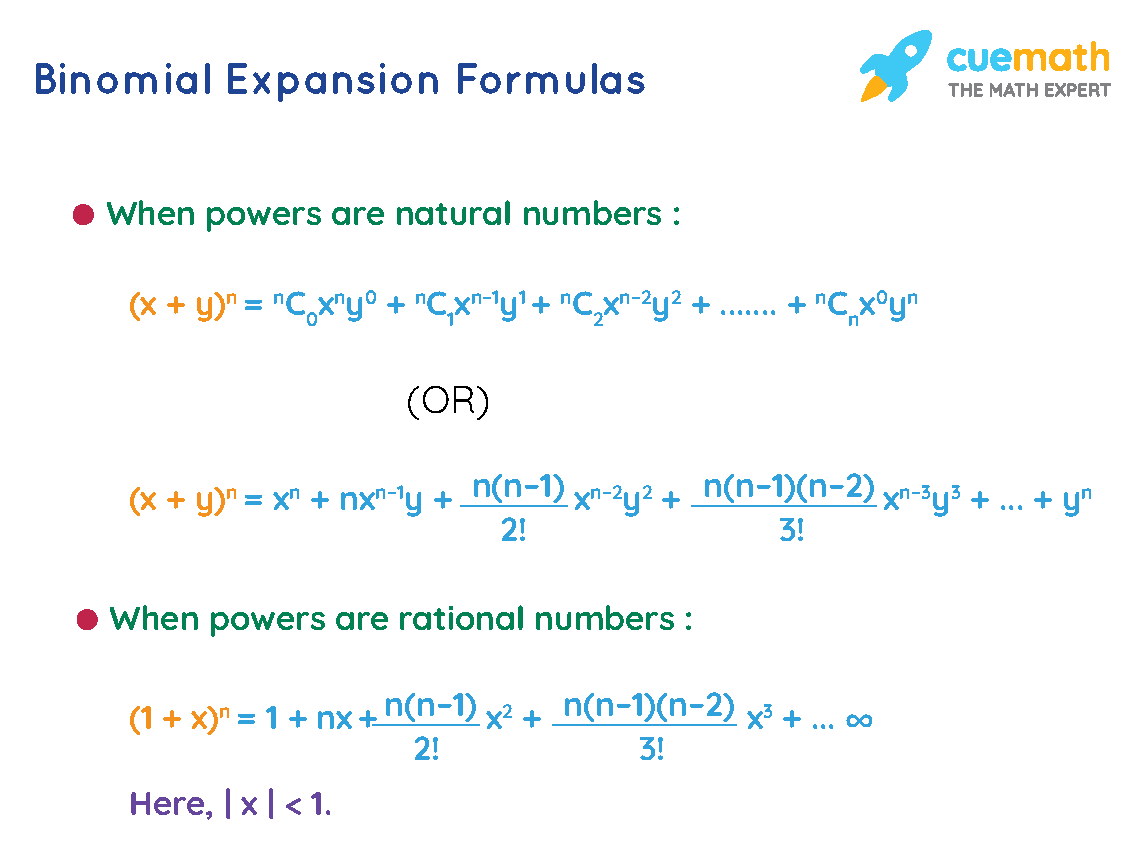
Binomial Expansion Formulas Derivation Examples

Expand Expand Terms Multiply Polynomials With Step By Step Math Problem Solver

Is There A Shortcut To Expand The Equation X Y Quora

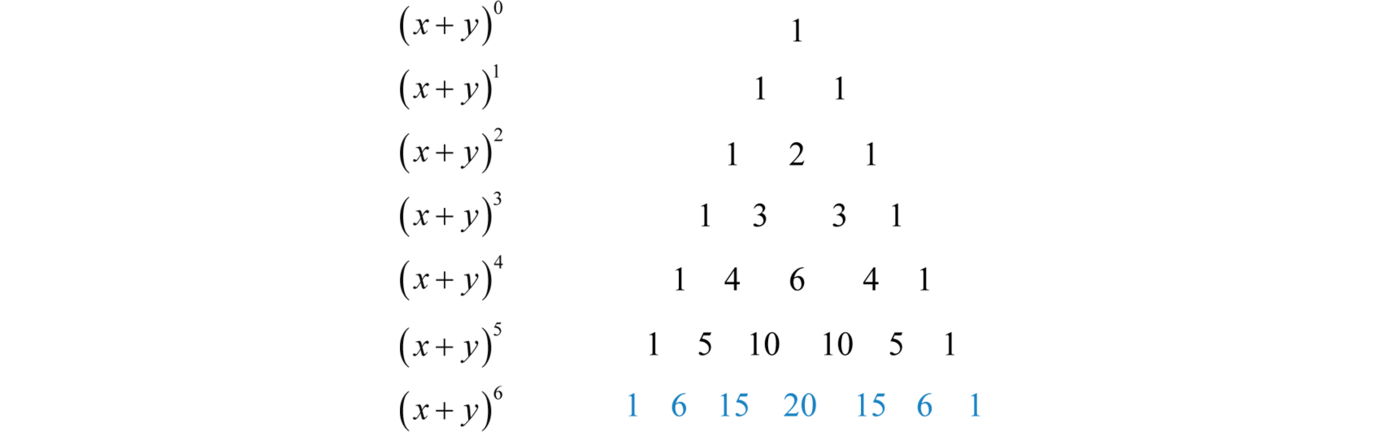
Binomial Theorem

Learn Algebraic Identity Of X Y And X Y In 3 Minutes

Lesson Worksheet Pascal S Triangle And The Binomial Theorem Nagwa

X Y 2 Expand Aoierrico

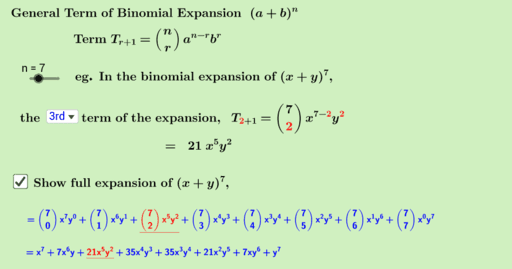
General Term Of Binomial Expansion Geogebra

Find The Expansion Of The Following X Y 1 Whole Cube Maths Expansions Meritnation Com

Binomial Theorem Wikipedia Republished Wiki 2

Learn Algebraic Identity Of X Y And X Y In 3 Minutes

Using The Binomial Theorem College Algebra

Learn Algebraic Identities Of X Y And X Y In 3 Minutes

How Do You Use The Binomial Theorem To Expand X Y 5 Socratic

Expand 1 X Y 3 3 Solve It Fastly Brainly In

Learn Algebraic Identity Of X Y And X Y In 3 Minutes

Is There A Shortcut To Expand The Equation X Y Quora

Expand 3x Y 5 Using The Binomial Theorem Mathsgee Q A Network

2

Ex 2 5 6 Write The Following Cubes In Expanded Form I 2x 1 3

A Lesson In Making Use Of Structure From With Jmccalla1 Experiments In Learning By Doing

9 5 The Binomial Theorem

How Do You Expand The Binomial X Y 5 Socratic

The Binomial Theorem Boundless Algebra

2

2 A Simplify By Collecting Like Terms 1 4x 3 Gauthmath

X Y 2 3 Find The Expansion Of The Following Brainly In

Expand Using Formula X Y 3 Brainly In

Binomial Expansion Binomial Expansions Copyright C By Houghton Mifflin Company Inc All Rights Reserved 2 The Binomial Theorem Provides A Useful Method Ppt Download

Section 8 5 The Binomial Theorem In This Section You Will Learn Two Techniques For Expanding A Binomial When Raised To A Power The First Method Is Called Ppt Download

Solved Question 2 46 Marks 2 1 Expand And Simplify The Chegg Com

Binomial Theorem Wikipedia

A Quick And Efficient Way To Expand Binomials Ppt Download

Binomial Expansion Made Easy Expand X Y Algebra Youtube

Section 8 5 The Binomial Theorem Ppt Download

Factorials To Binomial Theorem

Expand X Y 3 Sarthaks Econnect Largest Online Education Community

2

Expand X Y 3 Solved

Example 7 Find Coefficient Of X6y3 In Expansion X 2y 9

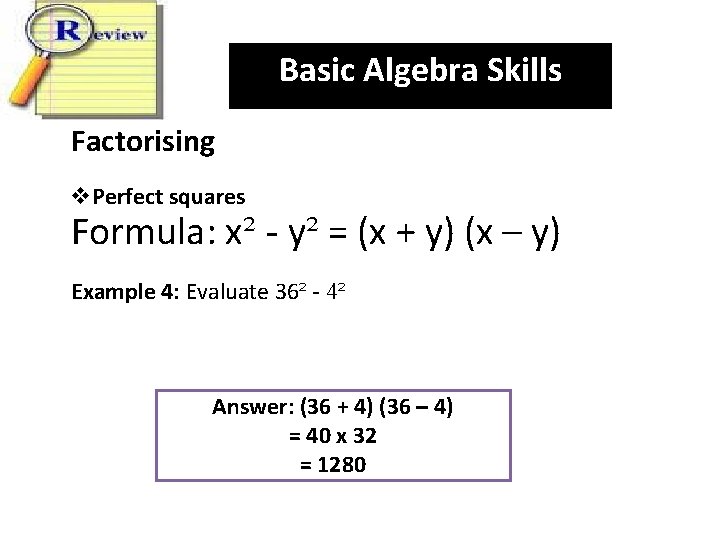
Basic Algebra Skills Factorising Add Brackets Expand This

How Do You Expand X 3 5 Using Pascal S Triangle Socratic

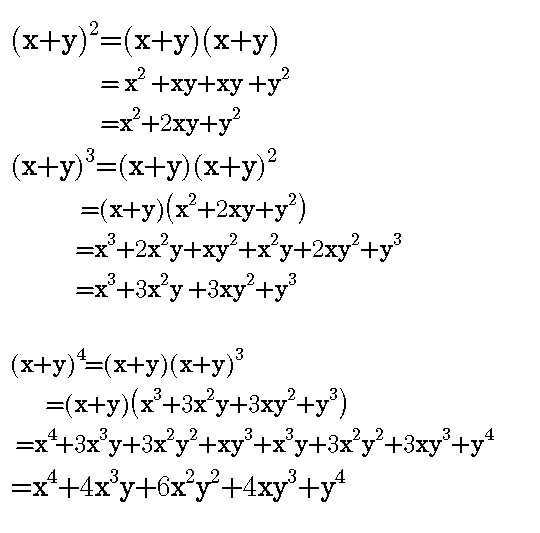
Worked Examples On Binomial Expansion Steemit

Expand The Function By The Taylor Formula In The Vicinity Of The Point Mathematics Stack Exchange

Expand Expand Terms Multiply Polynomials With Step By Step Math Problem Solver

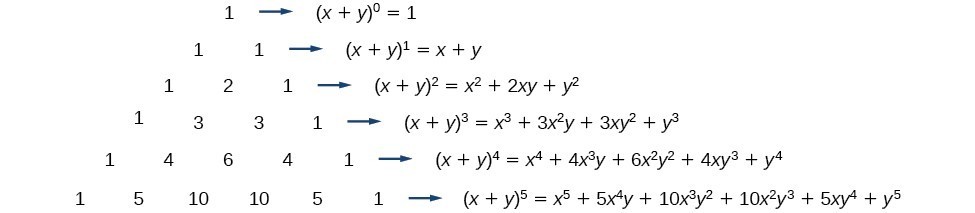
Using The Binomial Theorem College Algebra

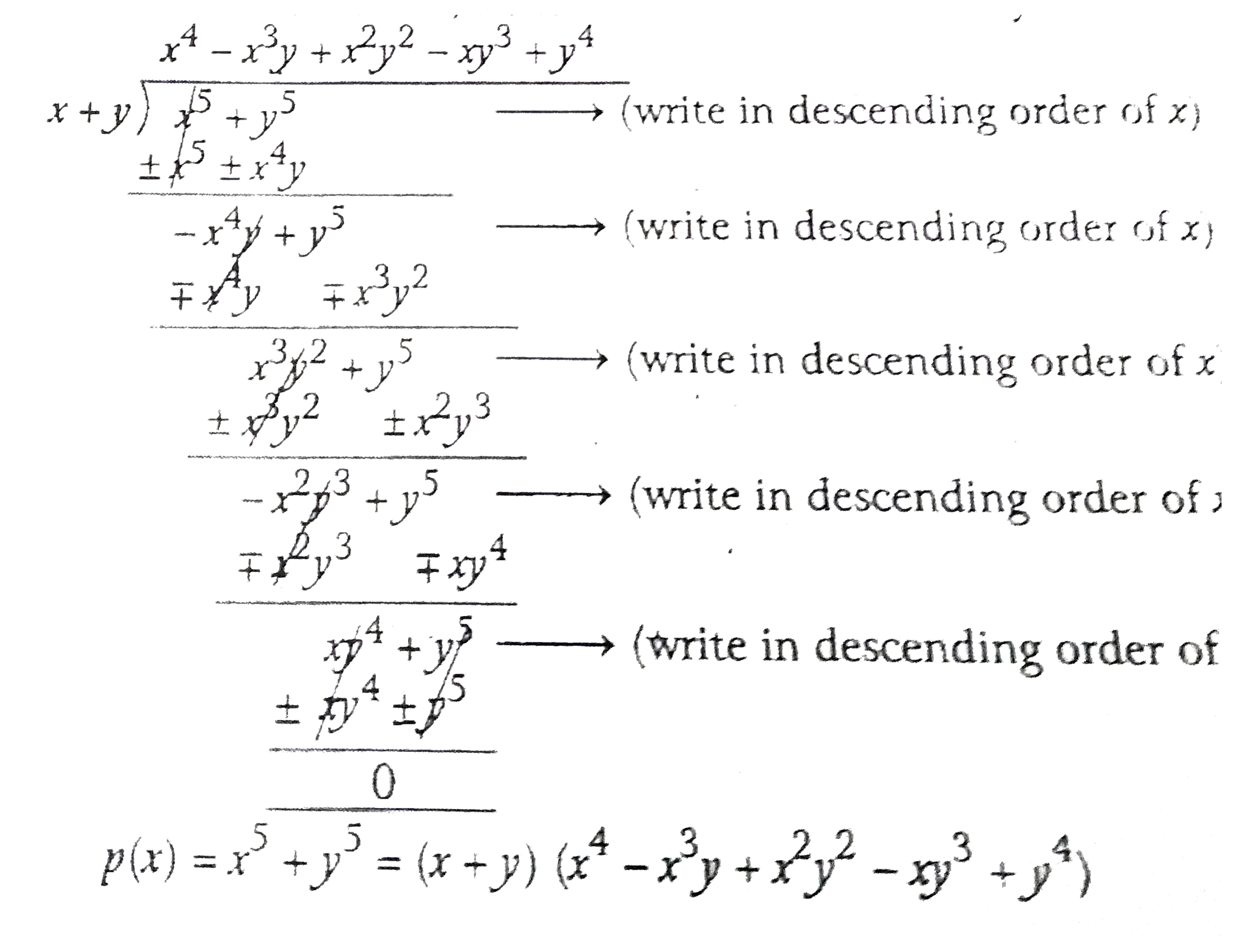
X Y 2 Expand Aoierrico

ベスト X5 Y5 Expand シモネタ

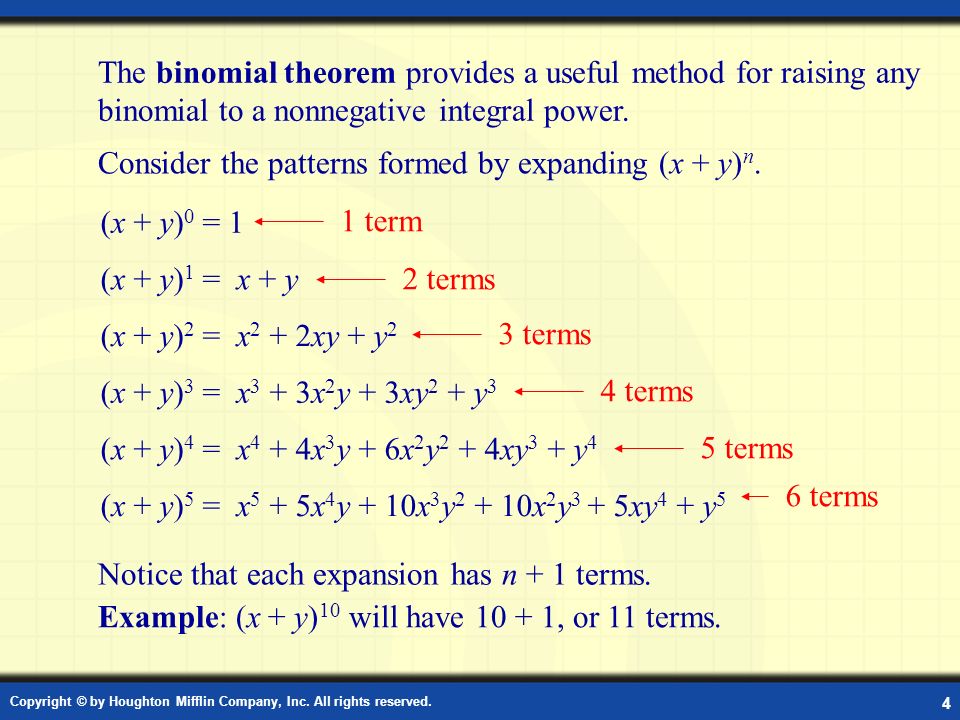
8 5 The Binomial Theorem Warm Up Copyright C By Houghton Mifflin Company Inc All Rights Reserved 2 X Y 3 X 3 3x 2 Y 3xy 2 Y Ppt Download

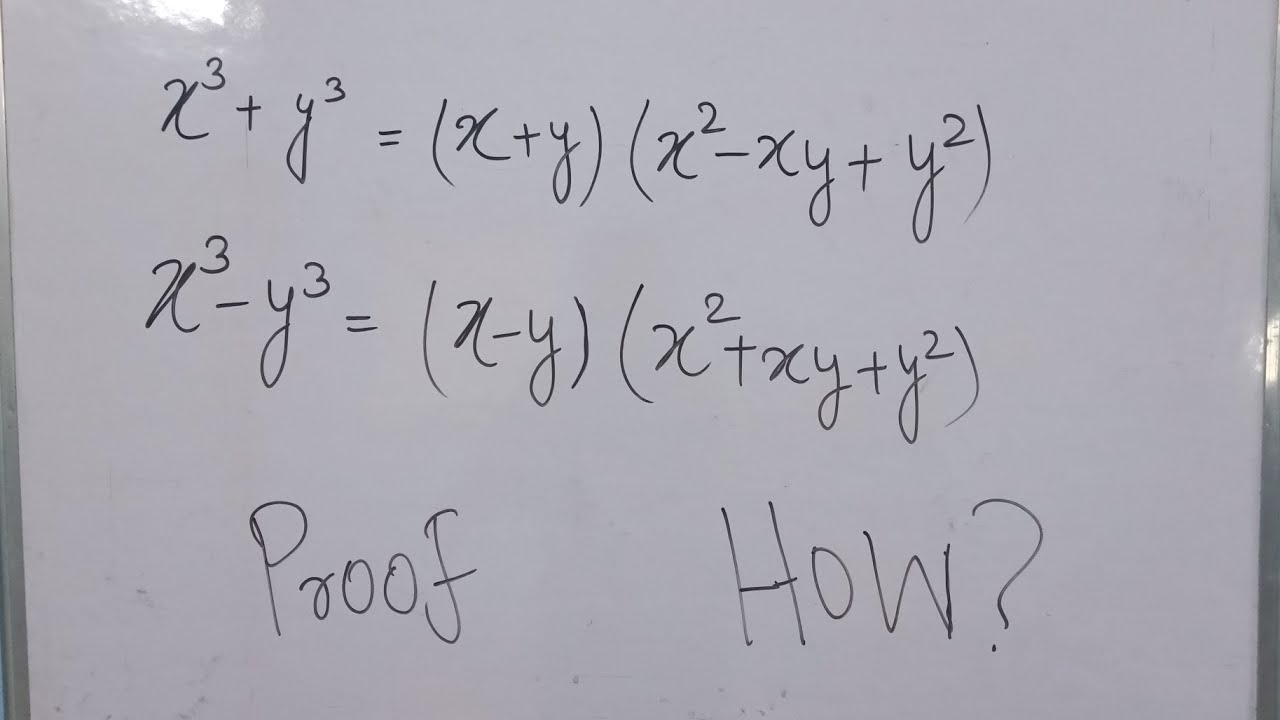
X 3 Y 3 X 3 Y 3 Formula Proof Youtube

1

Don T Understand Why This Binomial Expansion Is Not Valid For X 1 Mathematics Stack Exchange

Understanding Binomial Expansion Mathematics Stack Exchange

1

Binomial Expansion Christober S Technical Weblog

Example 22 I Write The Cubes In Expanded Form 3a 4b 3

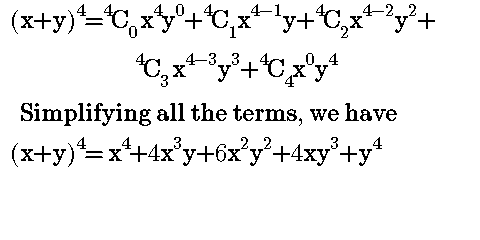
Worked Examples On Binomial Expansion Steemit

Taylor S Series Expansion An Overview Sciencedirect Topics

How To Expand Using The Identity X Y 3 X3 Y3 3x2y 3xy2 Youtube

Conic Section From Expanded Equation Ellipse Video Khan Academy

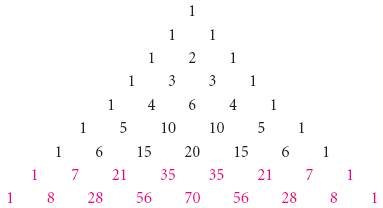
The Binomial Theorem Binomial Expansions Using Pascal S Triangle Subsets

X Y 2 Expand Aoierrico

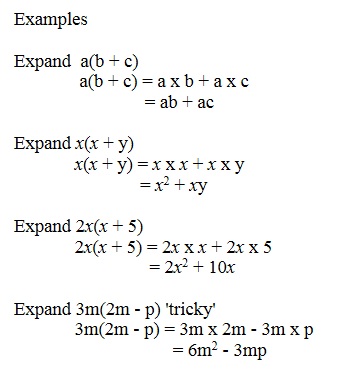
Algebra 5 Expanding Simplifying Mathtec

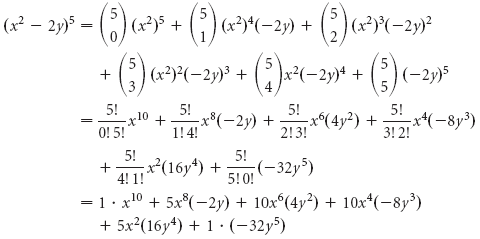
The Binomial Theorem Binomial Expansions Using Pascal S Triangle Subsets

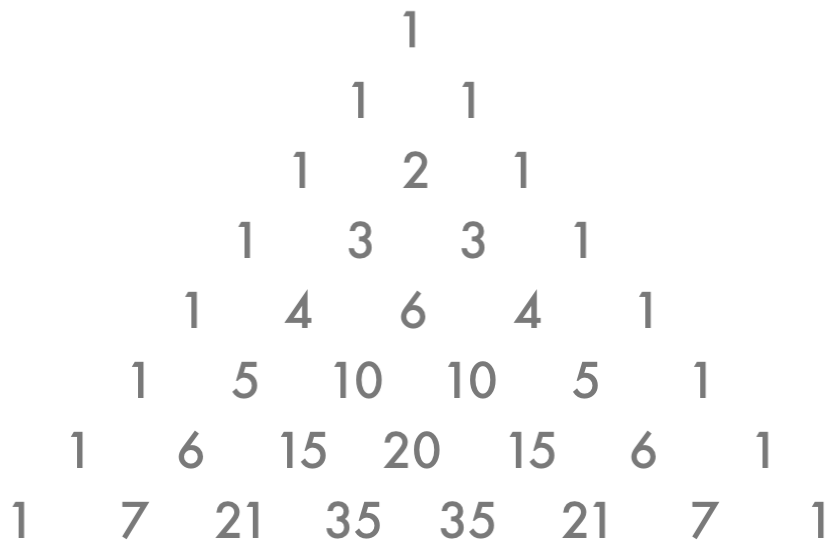
How Do You Expand X Y 6 Using Pascal S Triangle Socratic